Kruskal重构树

  学习笔记12 分钟读完 (大约1816个字)0次访问

浅谈重构树

Kruskal 重构树

简介

Kruskal重构树其实就是在Kruscal算法进行的过程中，每一次加边都会合并两个集合，我们可以新建一个点，将这个点的点权设为新加的边的权值，同时将两个集合的根节点分别设为新建的点的左儿子和右儿子。然后将两个集合和新建点合并成一个集合。将新建点设为根。

这样建成的一棵二叉树就是Kruskal重构树。

如：

此图的Kruskal重构树如下：

性质

  • 该树满足二叉堆的性质；
  • 这颗二叉树的节点个数为2n−12n-1 ，深度最大为nn;
  • 重构树中代表原树中的点的节点全是叶子节点，其余节点都代表了一条边的边权；
  • 原图中两个点之间的所有简单路径上最大边权的最小值 = Kruskal 重构树上两点之间的 LCA 的权值。

因为在加边的时候所有的边都是已经排序过的所以符合二叉堆的性质。

又因为二叉堆的性质所以Kruskal 重构树上两点之间的 LCA 的权值即为原图中两个点之间的所有简单路径上最大边权的最小值。

若求两个点之间的所有简单路径上最小边权的最大值，要建最大生成树。

构造

首先对边排序

然后使用并查集辅助加边：

每新建一条边时，新建一个点，设新建点的权值为新加边的边权，合并集合。

代码：

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
void kruskal()
{
    sort(e+1,e+m+1,cmp);
    for(int i=1;i<=n*2;i++)f[i]=i;// 2n-1个节点
    for(int i=1;i<=m;i++)
    {
        if(cnt==2*n-1)break;
        int x=Find(e[i].u),y=Find(e[i].v);
        if(x==y)continue;
        cnt++;
        f[x]=f[y]=cnt;
        tree[cnt].push_back(y);
        tree[cnt].push_back(x);
        w[cnt]=e[i].c;
    }
    return;
}

时间复杂度O(nlog2n)O(nlog_2n)。

应用

Kruskal重构树能够更快有效解决一些静态的树剖问题，而且复杂度还很优秀。

luogu U92652 【模板】Kruskal重构树

一道板子题（注意建成的应是一个森林）。

代码：

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
#include<bits/stdc++.h>
using namespace std;
const int Max=300010;
int n,m,q,cnt;
struct node{
    int u,v,c;
}e[Max];
int f[Max*2],w[Max*2],lg[Max*2];
int depth[Max*2],fa[Max*2][30];
vector<int> mp[Max*2];
int cmp(node x,node y)
{
    return x.c<y.c;
}
int Find(int x)
{
    if(x==f[x])return x;
    return f[x]=Find(f[x]);
}
void kruskal()
{
    for(int i=1;i<=n*2;i++)f[i]=i;
    sort(e+1,e+m+1,cmp);
    for(int i=1;i<=m;i++)
    {
        int x=Find(e[i].u),y=Find(e[i].v);
        if(x==y)continue;
        cnt++;
        f[x]=cnt;f[y]=cnt;
        w[cnt]=e[i].c;
        mp[cnt].push_back(x);
        mp[cnt].push_back(y);
    }
    return;
}
void dfs(int now,int father)
{
    depth[now]=depth[father]+1;
    fa[now][0]=father;
    for(int i=1;i<=lg[depth[now]];i++)
        fa[now][i]=fa[fa[now][i-1]][i-1];
    for(int i=0;i<mp[now].size();i++)
    {
        int x=mp[now][i];
        if(depth[x])continue;
        dfs(x,now);
    }
    return;
}
int LCA(int x,int y)
{
    if(Find(x)!=Find(y))return -1;
    if(depth[x]<depth[y])swap(x,y);
    while(depth[x]>depth[y])
        x=fa[x][lg[depth[x]-depth[y]]];
    if(x==y)return w[x];
    for(int i=lg[depth[x]];i>=0;i--)
    {
        if(fa[x][i]==fa[y][i])continue;
        x=fa[x][i];
        y=fa[y][i];
    }
    return w[fa[x][0]];
}
int main()
{
    scanf("%d%d%d",&n,&m,&q);
    cnt=n;
    for(int i=1;i<=m;i++)
        scanf("%d%d%d",&e[i].u,&e[i].v,&e[i].c);
    kruskal();
    for(int i=1;i<=n;i++)
        lg[i]=lg[i-1]+(i==1<<(lg[i-1]+1));
    for(int i=1;i<=cnt;i++)
    {
        if(f[i]!=i)continue;
        if(depth[f[i]])continue;
        dfs(f[i],0);
    }
    for(int i=1;i<=q;i++)
    {
        int x,y;scanf("%d%d",&x,&y);
        printf("%d\n",LCA(x,y));
    }
    return 0;
}

luogu P1967 货车运输

其实该问题可以转换为求xx号城市到yy号城市所有简单路径上小边权的最大值（注意该图建成的为一个森林）。

代码：

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
#include<bits/stdc++.h>
using namespace std;
const int maxn=1e4+10;
const int maxm=5e4+10;
int n,m,q,cnt;
struct node{
    int u,v,c;
}e[maxm];
int f[maxn*2],depth[maxn*2],father[maxn*2][30];
int lg[maxn*2],w[maxn*2];
vector<int> mp[maxn*2];
int cmp(node x,node y)
{
    return x.c>y.c;
}
int Find(int x)
{
    if(x==f[x])return x;
    return f[x]=Find(f[x]);
}
void kruscal()
{
    for(int i=1;i<=n*2;i++)f[i]=i;
    sort(e+1,e+m+1,cmp);
    for(int i=1;i<=m;i++)
    {
        int x=Find(e[i].u),y=Find(e[i].v);
        if(x==y)continue;
        cnt++;
        f[x]=f[y]=cnt;w[cnt]=e[i].c;
        mp[cnt].push_back(x);
        mp[cnt].push_back(y);
    }
    return;
}
void dfs(int now,int fa)
{
    depth[now]=depth[fa]+1;
    father[now][0]=fa;
    for(int i=1;i<=lg[depth[now]];i++)
        father[now][i]=father[father[now][i-1]][i-1];
    for(int i=0;i<mp[now].size();i++)
    {
        int x=mp[now][i];
        if(depth[x])continue;
        dfs(x,now);
    }
    return;
}
int LCA(int x,int y)
{
    if(Find(x)!=Find(y))return -1;
    if(depth[x]<depth[y])swap(x,y);
    while(depth[x]>depth[y])
        x=father[x][lg[depth[x]-depth[y]]];
    if(x==y)return w[x];
    for(int i=lg[depth[x]];i>=0;i--)
    {
        if(father[x][i]==father[y][i])continue;
        x=father[x][i];y=father[y][i];
    }
    return w[father[x][0]];
}
int main()
{
    scanf("%d%d",&n,&m);
    for(int i=1;i<=m;i++)
        scanf("%d%d%d",&e[i].u,&e[i].v,&e[i].c);
    cnt=n;
    kruscal();
    for(int i=1;i<=n;i++)
        lg[i]=lg[i-1]+(i==1<<(lg[i-1]+1));
    for(int i=1;i<=cnt;i++)
    {
        if(f[i]!=i)continue;
        if(depth[f[i]])continue;
        dfs(f[i],0);
    }
    scanf("%d",&q);
    for(int i=1;i<=q;i++)
    {
        int x,y;scanf("%d%d",&x,&y);
        printf("%d\n",LCA(x,y));
    }
    return 0;
}

luogu P4768 [NOI2018] 归程

这道题主要在于求最小的步行路程，不用考虑开车所经过的路程。

所以我们只需要两点之间路径上的边权最小值最大即可，设这个值为hh,若h>Sh>S,则步行路程为零，若h≤Sh\le S，我们便需要去判断在哪里下车。

在Kruskal重构树中，任意节点的子树节点都是可以相互抵达的，所以我们可以用树上倍增去求出，这棵树上大于水位线的最小值，然后输出这个节点子树中，到达终点的最短路的最小值。

最短路+树上倍增+Kruskal重构树。

代码：

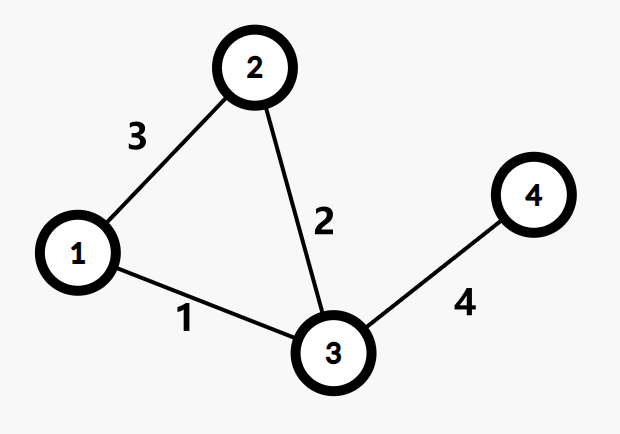
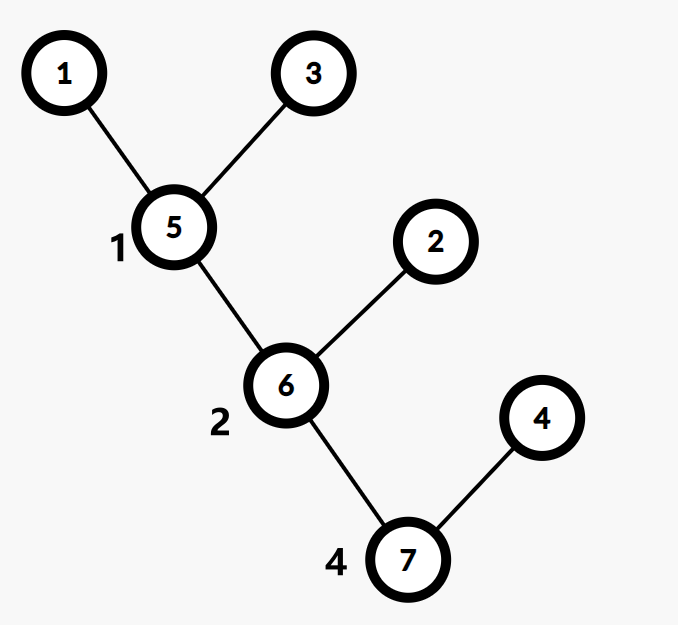
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122
123
124
125
126
127
128
129
130
131
132
133
134
135
136
137
138
139
140
141
#include<bits/stdc++.h>
#define ll long long
using namespace std;
const int maxn=2e5+10;
const int maxm=4e5+10;
const ll INF=5147483647;
int T,n,m,q,k,s,cnt;ll lastans;
int f[maxn*2],lg[maxn];
int depth[maxn*2],fa[maxn*2][31];
struct node{
    int u,v,l,a;
}e[maxm];
struct road{
    int to,cost;
};
struct power{
    ll w,l;
}p[maxn*2];
vector<road> mp[maxn];
vector<int> tree[maxn*2];
typedef pair<ll,int> Pair;
void _clean()
{
    memset(f,0,sizeof(f));
    memset(depth,0,sizeof(depth));
    for(int i=0;i<=cnt;i++)
        for(int j=0;j<=30;j++)
            fa[i][j]=0;
    for(int i=1;i<=n;i++)mp[i].clear();
    for(int i=1;i<=cnt;i++)tree[i].clear();
    cnt=0;lastans=0;
}
void makelog()
{
    for(int i=1;i<=maxn;i++)
        lg[i]=lg[i-1]+(i==1<<(lg[i-1]+1));
    return;
}
bool cmp(node x,node y)
{
    return x.a>y.a;
}
int Find(int x)
{
    if(x==f[x])return x;
    return f[x]=Find(f[x]);
}
void kruscal()
{
    sort(e+1,e+m+1,cmp);
    for(int i=1;i<=n*2;i++)f[i]=i;
    for(int i=1;i<=m;i++)
    {
        if(cnt==2*n-1)break;
        int x=Find(e[i].u),y=Find(e[i].v);
        if(x==y)continue;
        cnt++;
        f[x]=f[y]=cnt;
        tree[cnt].push_back(y);
        tree[cnt].push_back(x);
        p[cnt].w=e[i].a;
    }
    for(int i=1;i<=cnt;i++)p[i].l=INF;
    return;
}
void dij()
{
    priority_queue<Pair,vector<Pair>,greater<Pair> > q;
    bool vis[maxn];ll dis[maxn];
    memset(vis,false,sizeof(vis));
    for(int i=1;i<=n;i++)dis[i]=INF;
    dis[1]=0;
    q.push(make_pair(0,1));
    while(!q.empty())
    {
        int x=q.top().second;
        int c=q.top().first;
        q.pop();
        if(vis[x])continue;
        vis[x]=true;
        for(int i=0;i<mp[x].size();i++)
        {
            if(dis[mp[x][i].to]>dis[x]+mp[x][i].cost)
            {
                dis[mp[x][i].to]=dis[x]+mp[x][i].cost;
                q.push(make_pair(dis[mp[x][i].to],mp[x][i].to));
            }
        }
    }
    for(int i=1;i<=n;i++)p[i].l=dis[i];
    return;
}
void dfs(int now,int father)
{
    depth[now]=depth[father]+1;
    fa[now][0]=father;
    for(int i=1;i<=lg[depth[now]];i++)
        fa[now][i]=fa[fa[now][i-1]][i-1];
    for(int i=0;i<tree[now].size();i++)
    {
        dfs(tree[now][i],now);
        p[now].l=min(p[now].l,p[tree[now][i]].l);// 更新最短路最小值
    }
    return;
}
ll ask(int x,int y)
{
    for(int i=lg[depth[x]];i>=0;i--)
        if(p[fa[x][i]].w>y)x=fa[x][i];
    return p[x].l;
}
int main()
{
    makelog();
    scanf("%d",&T);
    while(T--)
    {
        scanf("%d%d",&n,&m);
        cnt=n;
        for(int i=1;i<=m;i++)
        {
            scanf("%d%d%d%d",&e[i].u,&e[i].v,&e[i].l,&e[i].a);
            mp[e[i].u].push_back((road){e[i].v,e[i].l});
            mp[e[i].v].push_back((road){e[i].u,e[i].l});
        }
        kruscal();
        dij();
        dfs(cnt,0);
        scanf("%d%d%d",&q,&k,&s);
        for(int i=1;i<=q;i++)
        {
            int x,y;scanf("%d%d",&x,&y);
            int vx=(x+k*lastans-1)%n+1;
            int py=(y+k*lastans)%(s+1);
            lastans=ask(vx,py);
            printf("%lld\n",lastans);
        }
        _clean();
    }
    return 0;
}

完结撒花~~

Kruskal重构树

https://jekyll-y.github.io/2022/06/13/Kruskal重构树/

作者

Jekyll_Y

发布于

2022-06-13

更新于

2023-03-02

许可协议

#

评论

Follow

目录

×