真的是超详细的计算几何全家桶哦!ヾ(≧▽≦*)o
upd:2022-7-25 又进行了一些修修补补,内容更多了,但我还是蒟蒻。
另外这篇文章的代码过于恶臭,请见谅。
计算几何
前置知识
可以在二维生物和三维生物之间自由转换
前言
计算几何的话估计很久就已经不考了吧,但学一学的话,似乎也是无所谓有也无所谓无的吧很有好处的,(毕竟可以水题)。
计算几何
定义:对几何外形信息的计算机表示分析。(其实就是利用计算机建立数学模型解决几何问题。)
计算几何研究的对象是几何图形。早期人们对于图像的研究一般都是先建立坐标系,把图形转换成函数,然后用插值和逼近的数学方法,特别是用样条函数作为工具来分析图形,取得了可喜的成功。然而,这些方法过多地依赖于坐标系的选取,缺乏几何不变性,特别是用来解决某些大挠度曲线及曲线的奇异点等问题时,有一定的局限性。
二维计算几何基础
平面直角坐标系
平面直角坐标系其实就是笛卡尔坐标系,在计算几何中,我们经常会用到坐标表示,点和向量都是通过坐标来保存的。
极坐标系
极角坐标系,是指在平面内由极点,极轴和极径组成的坐标系。
其实极点就是对应平面直角坐标系中的原点,极轴对应的就是 x 正半轴(似乎不太严谨),利用一个有序点对 P(d,θ) 来表示一个点,d 就是对应的极径,θ 为与极轴的夹角,(其实和平面直角坐标系没啥区别)
向量及其运算
向量的基础知识

向量(也称为欧几里得向量、几何向量、矢量):既有大小又有方向的量称为向量。在数学中研究的向量为自由向量,即只要不改变它的大小和方向,起点和终点可以任意平行移动的向量。记 a 或a。
有向线段:带有方向的线段称为有向线段。有向线段三要素:起点,方向,长度,有了三要素,终点就唯一确定。我们用有向线段表示向量。
向量的模:有向线段 AB 的长度称为向量的模,其实就是向量的大小。即为 ∣AB∣ 或 ∣a∣ 。
零向量:模为零的向量。零向量的方向任意。
单位向量:模为 1 的向量称为该方向上的单位向量。
平行向量:方向相同或相反的两个非零向量。记作 a∥b 。对于多个互相平行的向量,可以任作一条直线与这些向量平行,那么任一组平行向量都可以平移到同一直线上,所以平行向量又叫共线向量。
滑动向量:沿着直线作用的向量称为滑动向量。
固定向量:作用于一点的向量称为固定向量。
相等向量:模相等且方向相同的向量。
相反向量:模相等且方向相反的向量。
向量的夹角:已知两个非零向量 a,b, 作 OA=a,OB=b , 那么向量 a 与向量 b 的夹角。记作:$\left\langle a, b \right \rangle $ ,显然当 $\theta=0 $ 时,两向量同向, θ=π 时两向量反向,θ=2π 时,两向量垂直,记作 a⊥b 。并且我们规定 θ∈[0,π] 。
注意:平面向量具有方向性,我们并不能比较两个向量的大小(但可以比较两向量的模长)。但是两个向量可以相等。
向量的运算
设 a=(x1,y1), b=(x2,y2)。
加减法
既然向量具有平移不变性,那么 a+b 就是将两条有向线段相连,即: a+b=(x1+x2,y1+y2)。
那向量的减法 a−b,其实就是 a+(−b), 即 a−b=(x1−x2,y1−y2)。
向量的加减法是满足以下法则的:
- 三角形法则:若要求和的向量首尾顺次相连,那么这些向量的和为第一个向量的起点指向最后一个向量的终点;
- 平行四边形法则:若要求和的两个向量共起点,那么它们的和向量为以这两个向量为邻边的平行四边形的对角线,起点为两个向量共有的起点,方向沿平行四边形对角线方向。
所以说向量的加减法就有了几何意义,并且满足交换律和结合律。
数乘
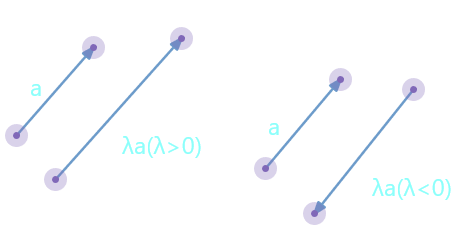
规定 ⌈实数λ与向量a的积⌋ 为一个向量,这种运算就是数乘。记作 λa , 并且满足以下性质:
- ∣λa∣=∣λ∣∣a∣;
- 当 λ>0 时,λa 与 a 同向,当 λ=0 时,λa=0,当 λ<0 时,λa 与 a 方向相反。
λ(μa)=(λμ)a(λ+μ)a=λa+μaλ(a+b)=λa+λb(−λ)a=−(λa)=−λ(a)λ(a−b)=λa−λb
(向量的数乘其实就是对向量进行放缩)
点积
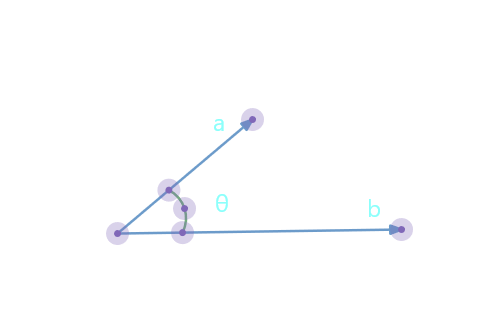
向量的点积也叫数量积、内积,向量的点积表示为 a⋅b, 是一个实数。计算式为:
a⋅b=∣a∣∣b∣cosθ(θ=⟨a,b⟩)(θ表示a,b的夹角)
三角形恒等变换的推导:

∵a⋅b=∣a∣∣b∣cosθ∴a⋅b=(x1)2+(y1)2⋅(x2)2+(y2)2⋅cosθ∵cosθ=cos(α−β)=cosαcosβ+sinαsinβ∵cosα=(x1)2+(y1)2x1,sinα=(y1)2+(y1)2x1cosβ=(x2)2+(y2)2x2,sinβ=(x2)2+(y2)2y2∴整理代入得:a⋅b=x1x2+y1y2
同时点积是满足交换律,结合律和分配律的。
叉积
也叫矢量积,外积。几何意义是两向量由平行四边形法则围成的面积。叉积是一个向量,垂直于原来两个向量所在的平面。(根据叉乘的模是平行四边形的面积我们可以想象,叉乘的结果是一个有方向的面,而面的方向平行于面的法线,所以面的方向垂直于面上任何一个向量),即:
∣a×b∣=∣a∣∣b∣sin⟨a,b⟩
(upd:这里的叉积定义感觉不太严谨,毕竟在数学中一般定义 ⟨a,b⟩∈[0,π], 这里显然 ∈[0,2π],但这可以帮助我们推出坐标表示下的公式)
并且,a×b=(x1y2−x2y1) 。
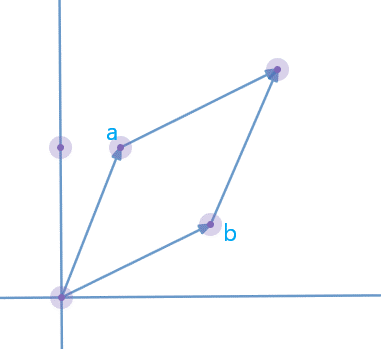
叉积是一个有向面积:
- a×b=0,等价于 a,b,共线(可以反向);
- a×b>0,b 在 a 左侧;
- a×b<0,b 在 a 右侧。
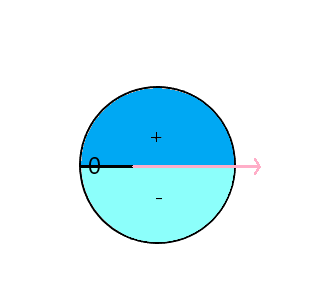
需要注意的是 a×b=b×a,只是绝对值相等。
判断两向量共线
两个非零向量 a 与 b 共线 ⟺ 有唯一实数 λ,使得 b=λa。由向量的数乘即可得证。
推论:如果 l 为已经过点 A 且平行于已知非零向量 a 的直线,那么对空间任一点 O,点 P 在直线 l 上的充要条件是存在实数 t ,满足等式:OP=OA+ta。
其中向量 a 叫做直线 l 的方向向量。
基本定理和坐标表示
平面向量基本定理
平面向量基本定理:两个向量 a 和 b 不共线的充要条件是,对于和向量 a ,b 共面的任意向量 p,有唯一实数对 (x,y) 满足 p=xa+yb。
证明(非常简单):
对于平面上的任意向量可以沿指定方向被分解为任意两个向量,平面上的任意两个向量可以沿指定方向合成任意指定向量。对于有唯一实数对我们可以用反证法:假设有两个及以上的实数对满足要求为(m,n)∴xa+yb=ma+nb∴(x−m)a=(n−y)b∵a和b不共线∴x=m,n=y与假设矛盾所以结论成立证毕
在同一平面内的两个不共线的向量称为基底。
如果基底相互垂直,那么我们在分解的时候就是对向量正交分解。
平面向量的坐标表示
如果取与横轴与纵轴方向相同的单位向量 i,j 作为一组基底,根据平面向量基本定理,平面上的所有向量与有序实数对 (x,y) 一一对应。
而有序数对 (x,y) 与平面直角坐标系上的点一一对应,那么我们作 OP=p ,那么终点 P(x,y) 也是唯一确定的。由于我们研究的都是自由向量,可以自由平移起点,这样,在平面直角坐标系里,每一个向量都可以用有序实数对唯一表示。
坐标运算
平面向量线性运算
由平面向量的线性运算,我们可以推导其坐标运算,主要方法是将坐标全部化为用基底表示,然后利用运算律进行合并,之后表示出运算结果的坐标形式。
对于向量 a=(m,n) 和向量 b=(p,q) ,则有:
a+b=(m+n,n+q)a−b=(m−n,n−q)ka=(km,kn),kb=(kq,kq)
向量的坐标表示
已知两点 A(a,b),B(c,d) , 则 AB=(c−a,d−b)。
平移一点
将一点 P 沿一定方向平移某单位长度,只需要将要平移的方向和距离组合成一个向量,利用三角形法则,用 OP 加上这个向量即可,得到的向量终点即为平移后的点。
三点共线的判定
在平面上 A,B,C 三点共线的充要条件是:OC=λOB+(1−λ)OA,(O为平面内不与直线AC共线任意一点)。
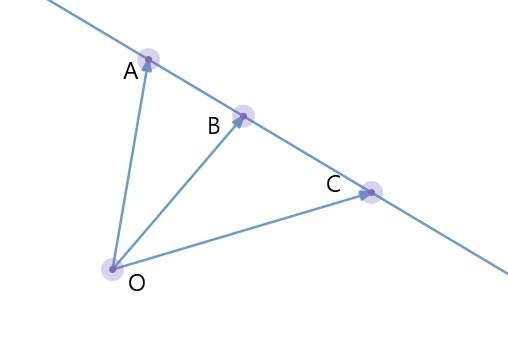
证明:
若点B与AC共线,由共线向量定理可知:AC=λAB∴AC=λAB⟺OC−OA=λ(OB−OA)⟺OC=(1−λ)OA+λOB证毕
三维计算几何基础
坐标表示
和平面直角坐标系差不多,就是变成了空间直角坐标系,加了条 z 轴,多了个右手定则和左手定则(也不常用),表示空间中的一个点的时候,用一个有序数对 (x,y,z) 即可。
向量 a(x,y,z) 的模长为:
∣a∣=x2+y2+z2
向量运算
数乘
并没有太大的变化,只不过是变成了三维坐标,设 a=(x,y,z), 有
ka=(kx,ky,kz)
点积
设 a=(x1,y1,z1),b=(x2,y2,z2),则
a⋅b=∣a∣∣b∣cos⟨a,b⟩
又可以表示为:
a⋅b=x1x2+y1y2+z1z2
是一个常数。
叉积
空间直角坐标系中向量 a 和向量 b 的叉积为:
a×b=(y1z2−y2z1,x2z1−x1z2,x1y2−x2y1)
结果是一个向量,另外可以用行列式表示,
设 c=a×b,c=(x3,y3,z3), 叉积结果为:
∣∣∣∣∣∣∣x3x1x2y3y1y2z3z1z2∣∣∣∣∣∣∣
将其展开即为 :
x3(y1z2−y2z1)+y3(x2z1−x1z2)+z3(x1y2−x2y1)
叉积结果即为 (y1z2−y2z1,x2z1−x1z2,x1y2−x2y1),
另外根据叉积的定义,在空间直角坐标系中叉积所代表的向量为两个进行叉积向量所在平面的法向量。
tips: 三阶行列式的展开
比如上面的
∣∣∣∣∣∣∣x3x1x2y3y1y2z3z1z2∣∣∣∣∣∣∣
将其第一,二列 copy 到第三列右边:
∣∣∣∣∣∣∣x3x1x2y3y1y2z3z1z2∣∣∣∣∣∣∣∣∣∣∣∣∣∣x3x1x2y3y1y2∣∣∣∣∣∣∣
用第 1, 2, 3 列的对角线的乘积和减去第 3, 4, 5 列的乘积和:
(x3y1z2+y3z1x2+z3x1y2)−(y3x1z2+x3z1y2+z3y1x2)
化简即上述结果,(算错可就寄了)
平面的表示
我们可以用平面上的一点 P(x,y,z) 和该平面的法向量 n(a,b,c) 来表示一个平面,满足 P⋅n=0 的点集即为该平面,可以推出,
Ax+By+Cz+D=0
为平面的一般式。
三维计算几何其实也没啥,就是升维打击。
凸包
二维凸包
凸多边形
凸多边形是指所有内角在 [0,π] 范围内的简单多边形。
凸包
对于在平面上的一个点集,凸包是能包含所有点的最小凸多边形。
其定义为:对于给定集合 D,所有包含 D 的凸集的交集 S 被称为 D 的凸包。
如:
凸包求法
对于平面上的一个点集,其凸包可以用分治,Graham−Scan,和 Andrew。
分治
对于分治算法解决凸包问题,递归求解,找到子问题的凸包,讲左右两个子集的凸包进进行合并即可,时间复杂度 (nlogn)。
Andrew
对于 Andrew 算法,主要流程:
-
首先将所有点以横坐标为第一关键字,纵坐标为第二关键字进行排序;
-
显然排序后最小的元素和最大的元素一定在凸包上,然后用单调栈维护上下凸壳;
-
因为上下凸壳所旋转的方向不同,我们首先升序枚举下凸壳,然后降序枚举上凸壳。
时间复杂度 (nlogn)。
Graham−Scan
主要介绍这种算法,更好写一些,首先我们先找出在最右下角的点,此时这个点一定在凸包上,然后我们从这个点开始逆时针旋转,同时用单调栈维护凸包上的点,每加入一个新点是判断改点是否会出现,该边在上一条边的“右边”,如果出现则删除上一个点。

【模板】二维凸包
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
| #include<bits/stdc++.h>
using namespace std;
const int maxn=1e5+10;
int n, top; double ans;
struct geometric{
double x,y,dss;
friend geometric operator + (const geometric a,const geometric b){return (geometric){a.x+b.x,a.y+b.y};}
friend geometric operator - (const geometric a,const geometric b){return (geometric){a.x-b.x,a.y-b.y};}
double dis(geometric a,geometric b){return sqrt((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y));}
double dot(geometric a1,geometric a2,geometric b1,geometric b2){return (a2.x-a1.x)*(b2.x-b1.x)+(a2.y-a1.y)*(b2.y-b1.y);}
double cross(geometric a1,geometric a2,geometric b1,geometric b2){return (a2.x-a1.x)*(b2.y-b1.y)-(a2.y-a1.y)*(b2.x-b1.x);}
};
geometric origin, data[maxn], st[maxn];
bool vis[maxn];
bool cmp(geometric a, geometric b)
{
geometric opt;
double tamp=opt.cross(data[1],a,data[1],b);
if(tamp>0)return true;
if(tamp==0&&opt.dis(data[1],a)<=opt.dis(data[1],b))return true;
return false;
}
geometric opt;
int main()
{
scanf("%d",&n);
for(int i=1;i<=n;i++)
{
scanf("%lf%lf",&data[i].x,&data[i].y);
if(i!=1&&data[i].y<data[1].y)
{
double tmp;
swap(data[1].y,data[i].y);
swap(data[1].x,data[i].x);
}
if(i!=1&&data[i].y==data[1].y&&data[i].x>data[1].x)
swap(data[1].x,data[i].x);
}
sort(data+2,data+n+1,cmp);
st[++top]=data[1];
for(int i=2;i<=n;i++)
{
while(top>1&&opt.cross(st[top-1],st[top],st[top],data[i])<=0)top--;
st[++top]=data[i];
}
st[++top]=data[1];
for(int i=1;i<top;i++)ans+=opt.dis(st[i],st[i+1]);
printf("%.2lf",ans);
return 0;
}
|
动态凸包
首先我们考虑这样一个问题:
两种操作:
- 向点集中添加一个点 (x,y);
- 询问点是否在凸包中。
首先我们对于一个动态的凸包,很明显在每次加入新点时不能再进行一次 Graham−Scan,否则时间复杂度不优。
那么我们就可以用一下方法:
对于平衡树我们可以直接用 STL 里的 set 去实现,需要用到迭代器。
Professor’s task
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
| #include<bits/stdc++.h>
#define it set<geometric>::iterator
#define eps 1e-8
using namespace std;
const int maxn=1e5+10;
int Sure(double x){return fabs(x)<eps?0:(x<0?-1:1);}
struct geometric{
double x,y;
geometric(double X=0,double Y=0):x(X),y(Y) {}
friend geometric operator + (const geometric a,const geometric b){return geometric(a.x+b.x,a.y+b.y);}
friend geometric operator - (const geometric a,const geometric b){return geometric(a.x-b.x,a.y-b.y);}
friend geometric operator * (const geometric a,double p){return geometric(a.x*p,a.y*p);}
friend geometric operator / (const geometric a,double p){return geometric(a.x/p,a.y/p);}
double dis(geometric a,geometric b){return sqrt((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y));}
double dot(geometric a1,geometric a2,geometric b1,geometric b2){return (a2.x-a1.x)*(b2.x-b1.x)+(a2.y-a1.y)*(b2.y-b1.y);}
double cross(geometric a1,geometric a2,geometric b1,geometric b2){return (a2.x-a1.x)*(b2.y-b1.y)-(a2.y-a1.y)*(b2.x-b1.x);}
double corner(geometric a1,geometric a2,geometric b1,geometric b2){return dot(a1,a1,b1,b2)/(dis(a1,a2)*dis(b1,b2));}
double area(geometric a1,geometric a2,geometric b1,geometric b2){return fabs(cross(a1,a2,b1,b2));}
double angle(geometric a){return atan2(a.y,a.x);}
geometric rotate_clockwise(geometric a,double theta){return geometric(a.x*cos(theta)-a.y*sin(theta),a.x*sin(theta)+a.y*cos(theta));}
geometric rotate_counterclockwise(geometric a,double theta){return geometric(a.x*cos(theta)+a.y*sin(theta),-a.x*sin(theta)+a.y*cos(theta));}
}opt,d[maxn],origin;
bool operator < (geometric a,geometric b){
a=a-origin;b=b-origin;
double ang1=atan2(a.y,a.x),ang2=atan2(b.y,b.x);
double l1=sqrt(a.x*a.x+a.y*a.y),l2=sqrt(b.x*b.x+b.y*b.y);
if(Sure(ang1-ang2)!=0)return Sure(ang1-ang2)<0;
else return Sure(l1-l2)<0;
}
int q,cnt;
set<geometric> S;
it Pre(it pos){if(pos==S.begin())pos=S.end();return --pos;}
it Nxt(it pos){++pos; return pos==S.end() ? S.begin():pos;}
bool Query(geometric key)
{
it pos=S.lower_bound(key);
if(pos==S.end())pos=S.begin();
return Sure(opt.cross(*(Pre(pos)),key,*(Pre(pos)),*(pos)))<=0;
}
void Insert(geometric key)
{
if(Query(key))return;
S.insert(key);
it pos=Nxt(S.find(key));
while(S.size()>3&&Sure(opt.cross(*(Nxt(pos)),*(pos),*(Nxt(pos)),key))>=0)
{
S.erase(pos);pos=Nxt(S.find(key));
}
pos=Pre(S.find(key));
while(S.size()>3&&Sure(opt.cross(*(Pre(pos)),*(pos),*(Pre(pos)),key))<=0)
{
S.erase(pos);pos=Pre(S.find(key));
}
}
int main()
{
scanf("%d",&q);
for(int i=1;i<=3;i++)
{
int opt;double x,y;scanf("%d%lf%lf",&opt,&x,&y);
d[++cnt]=geometric(x,y);origin.x+=x;origin.y+=y;
}
origin=origin/3.0;
for(int i=1;i<=3;i++)S.insert(d[i]);
for(int i=4;i<=q;i++)
{
int opt;double x,y;
scanf("%d%lf%lf",&opt,&x,&y);
d[++cnt]=geometric(x,y);
if(opt==1)Insert(d[cnt]);
else {
if(Query(d[cnt]))printf("YES\n");
else printf("NO\n");
}
}
return 0;
}
|
注意使用迭代器时不要越界,要和用指针一样小心!
三维凸包
对于 a(x1,y1) 和 b(x2,y2)
三维凸包的话,主要是空间几何坐标系中点积为:
a⋅b=x1x2+y1y2+z1z2
叉积为向量(法向量):
a×b=(y1z2−y2z1,x2z1−z2x1,x1y2−x2y1)
对于三维凸包的解法有很多种,介绍几种比较常用的吧。
增量法
主要是考虑加入一个点时如何维护凸包, 每加入一个点,从这个点“能看见的面”都不会是最终凸包的面,将这些不会成为答案的面删去就好了。
另外如果新加入的点在已经加入的面上,不用删除这个面。
首先先选取四个顶点,构成一个四面体,作为初始凸包,每加入一个点去在已经加入面中去找,从这个点能看到的面,将其删除,
关于存面的时候,同一条边会被使用两次,被顺时针使用一次,逆时针使用一次,可以对每条边打上标记,删掉不需要的面后对于只使用过一次的边按照其使用方向与新点建面,
存面的时候只需用到三个点就可以了,对于多个点共面的情况,因为我们用的是三个点来存面,最后的凸多边形,有可能会出现多个点共面的情况,导致将这个面分成多个小三角形,为了免去去重的一些操作,更好写一些,可以对点进行扰动,加一个不算大的误差。
时间复杂度 O(n2) 而且支持在线。
【模板】三维凸包
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
| #include<bits/stdc++.h>
#define eps 1e-10
#define double long double
using namespace std;
const int maxn=2010;
double randeps(){return (rand()/(double)RAND_MAX-0.5)*eps; }
struct geometric{
double x,y,z;
geometric(double X=0,double Y=0,double Z=0):x(X),y(Y),z(Z) {}
void shake(){x+=randeps(),y+=randeps(),z+=randeps();}
friend geometric operator + (const geometric a,const geometric b){return geometric(a.x+b.x,a.y+b.y,a.z+b.z);}
friend geometric operator - (const geometric a,const geometric b){return geometric(a.x-b.x,a.y-b.y,a.z-b.z);}
friend geometric operator * (const geometric a,double p){return geometric(a.x*p,a.y*p,a.z*p);}
friend geometric operator / (const geometric a,double p){return geometric(a.x/p,a.y/p,a.z/p);}
double dis(geometric a,geometric b){return sqrt((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y)+(a.z-b.z)*(a.z-b.z));}
double dot(geometric a1,geometric a2,geometric b1,geometric b2){return (a2.x-a1.x)*(b2.x-b1.x)+(a2.y-a1.y)*(b2.y-b1.y)+(a2.z-a1.z)*(b2.z-b1.z);}
geometric cross(geometric a1,geometric a2,geometric b1,geometric b2){geometric a=a2-a1,b=b2-b1;return geometric(a.y*b.z-b.y*a.z,b.x*a.z-a.x*b.z,a.x*b.y-b.x*a.y);}
}opt, origin, p[maxn];
struct plane{
int v[3];
plane(){v[0]=v[1]=v[2]=0;}
plane(int A,int B,int C){v[0]=A,v[1]=B,v[2]=C;}
geometric normal(){return opt.cross(p[v[0]],p[v[1]],p[v[0]],p[v[2]]);}
double area(){return fabs(opt.dis(normal(),origin))/2.0;}
}con[maxn<<1], h[maxn<<1];
int check(plane alpha, geometric vec){return opt.dot(origin, alpha.normal(), p[alpha.v[0]], vec)>0; }
int n, cnt, indx, l, vis[maxn][maxn]; double S;
int main()
{
srand(time(0));
scanf("%d",&n);
for(int i=1;i<=n;i++)
{
double x,y,z;
scanf("%Lf%Lf%Lf",&x,&y,&z);
p[++cnt]=geometric(x,y,z);
p[i].shake();
}
con[++indx]=plane(1,2,3);
con[++indx]=plane(3,2,1);
for(int i=4;i<=cnt;i++)
{
for(int j=1,res;j<=indx;j++)
{
res=check(con[j],p[i]);
if(!res)h[++l]=con[j];
for(int k=0;k<3;k++)
{
vis[con[j].v[k]][con[j].v[(k+1)%3]]=res;
}
}
for(int j=1;j<=indx;j++)
{
for(int k=0;k<3;k++)
{
int x=con[j].v[k],y=con[j].v[(k+1)%3];
if(vis[x][y]&&!vis[y][x])h[++l]=plane(x,y,i);
}
}
for(int j=1;j<=l;j++)con[j]=h[j];
indx=l,l=0;
}
for(int i=1;i<=indx;i++)S+=con[i].area();
printf("%0.3Lf",S);
return 0;
}
|
卷包裹法
其实就像是用一张纸去旋转包住凸包,碰到的第一个点作为凸包顶点,额不太会写。
时间复杂度 O(nh) ,h 为凸包点数,随机数据下能跑成 O(n)?
QuickHull
不会,挖坑。(也许不会补,比竟难写,又不用)
时间复杂度 O(nlogn) 好像是最快的。
一些例题:
[SHOI2012]信用卡凸包
[HAOI2011]防线修建
[HNOI2008]水平可见直线
[SDOI2013]保护出题人
旋转卡壳
读法
(其实我也不知道该怎么读,有 16 种读法)
凸多边形的切线
如果一条直线与凸多边形有交点,并且整个凸多边形都在这条直线的一侧,那么这条直线就是该凸多边形的一条切线。
对踵点
如果过凸多边形上两点作一对平行线,使得整个多边形都在这两条线之间,那么这两个点被称为一对对踵点。
凸多边形的直径
即凸多边形上任意两个点之间距离的最大值。直径一定会在对踵点中产生,如果两个点不是对踵点,那么两个点中一定可以让一个点向另一个点的对踵点方向移动使得距离更大。并且点与点之间的距离可以体现为线与线之间的距离,在非对踵点之间构造平行线,一定没有在对踵点构造平行线优,这一点可以通过平移看出。
旋转卡壳
先上一张比较标致的图,来对旋转卡壳有一个初步的了解:

对于实现旋转卡壳,我们首先可以先去求出凸包,然后去枚举每一条边,去找对踵点,即可找出凸包直径。时间复杂度 O(nlogn)
在旋转的时候用叉积对应的面积,来找对踵点就好了,因为在凸包上有一个单调性,在找对踵点的时候,实际上是 O(n) 的,建议好好思考一下。

注意,在旋转时为一个环,需要注意边界问题,要不要会收获血与泪的教训 (别问我怎么知道的)
【模板】旋转卡壳
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
| #include<bits/stdc++.h>
using namespace std;
const int maxn=5e4+10;
struct geometric{
double x,y,dss;
friend geometric operator + (const geometric a,const geometric b){return (geometric){a.x+b.x,a.y+b.y};}
friend geometric operator - (const geometric a,const geometric b){return (geometric){a.x-b.x,a.y-b.y};}
double dis(geometric a,geometric b){return sqrt((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y));}
double dot(geometric a1,geometric a2,geometric b1,geometric b2){return (a2.x-a1.x)*(b2.x-b1.x)+(a2.y-a1.y)*(b2.y-b1.y);}
double cross(geometric a1,geometric a2,geometric b1,geometric b2){return (a2.x-a1.x)*(b2.y-b1.y)-(a2.y-a1.y)*(b2.x-b1.x);}
double corner(geometric a1,geometric a2,geometric b1,geometric b2){return dot(a1,a1,b1,b2)/(dis(a1,a2)*dis(b1,b2));}
};
int n,top;double ans;
geometric d[maxn],opt,st[maxn];
bool cmp(geometric a,geometric b)
{
double tamp=opt.cross(d[1],a,d[1],b);
if(tamp>0)return true;
if(tamp==0&&opt.dis(d[1],a)<opt.dis(d[1],b))return true;
return false;
}
int main()
{
scanf("%d",&n);
for(int i=1;i<=n;i++)
{
scanf("%lf%lf",&d[i].x,&d[i].y);
if(i!=1&&d[i].y<d[1].y)
{
swap(d[1].y,d[i].y);
swap(d[1].x,d[i].x);
}
if(i!=1&&d[i].y==d[1].y&&d[i].x>d[1].x)
swap(d[1].x,d[i].x);
}
sort(d+2,d+n+1,cmp);
st[++top]=d[1];
for(int i=2;i<=n;i++)
{
while(top>1&&opt.cross(st[top-1],st[top],st[top],d[i])<=0)top--;
st[++top]=d[i];
}
st[++top]=d[1];
for(int i=2,j=3;i<=top;i++)
{
while(opt.cross(st[i-1],st[i],st[i-1],st[j])<opt.cross(st[i-1],st[i],st[i-1],st[j+1]))j==top-1?j=1:j++;
ans=max(ans,max(opt.dis(st[i],st[j]),opt.dis(st[i-1],st[j])));
}
printf("%d",int(ans*ans));
return 0;
}
|
一些例题
[HNOI2007]最小矩形覆盖
[SCOI2007]最大土地面积
[HNOI2008]水平可见直线
[ZJOI2008]瞭望塔
部分资料参考自洛谷日报
半平面交
半平面
平面内的一条直线把这个平面分成两部分,每一部分对这个平面来说,都叫做半平面。包括这条直线的半平面叫做闭半平面,否则叫做开半平面。
解析式为 Ax+By+C≥0 或 Ax+By+C<≤0 。
在计算几何中用向量表示,整个题统一以向量的左侧或右侧为半平面。

半平面交
半平面交就是多个半平面的交集。半平面交是一个点集。
它可以理解为向量集中每一个向量的右侧的交,或者是下面方程组的解。
{A1x+B1y+C1≥0A2x+B2y+C2≥0
多边形的核
如果一个点集中的点与多边形上任意一点的连线与多边形没有其他交点,那么这个点集被称为多边形的核。
把多边形的每条边看成是首尾相连的向量,那么这些向量在多边形内部方向的半平面交就是多边形的核。
求法
D&C 算法
该算法是基于分治思想的:
时间复杂度 (nlogn) 这个算法并不常用,主要介绍的是下面这个。
S&I 算法
该算法是在 2006 年有中国队队员朱泽园提出来的“排序增量法”。
假设给出 n 条直线,求这 n 条直线的左方半平面的交集:
注意事项!!!
在弹出不需要的交点时一定要先处理队尾再处理队首,因为在插入向量时,有可能是会把队首向量弹出的,此时如果先弹队首没回出现误删,
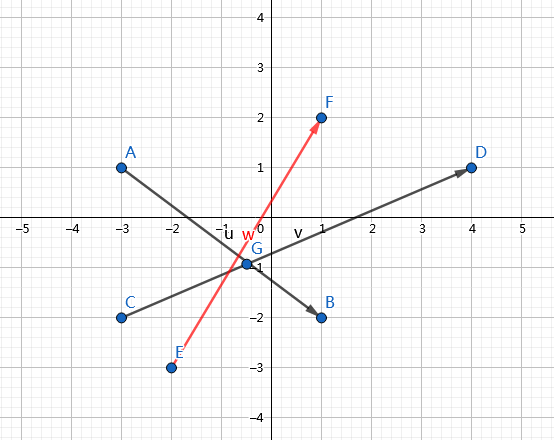
( AB 先入队)
此时我们插入 EF ,G 点肯定是要删去,但是如果我们先弹队首就会把可能会成为答案的 AB 删去,因为按逆时针排序,所以后插入的 CD 影响会更大一些,应先处理队尾。
【模板】半平面交
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
| #include<bits/stdc++.h>
#define eps 1e-8
using namespace std;
const int maxn=1010;
struct geometric{
double x,y;
geometric(double X=0,double Y=0):x(X),y(Y) {}
friend geometric operator + (const geometric a,const geometric b){return geometric(a.x+b.x,a.y+b.y);}
friend geometric operator - (const geometric a,const geometric b){return geometric(a.x-b.x,a.y-b.y);}
friend geometric operator * (const geometric a,double p){return geometric(a.x*p,a.y*p);}
friend geometric operator / (const geometric a,double p){return geometric(a.x/p,a.y/p);}
double dis(geometric a,geometric b){return sqrt((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y));}
double dot(geometric a1,geometric a2,geometric b1,geometric b2){return (a2.x-a1.x)*(b2.x-b1.x)+(a2.y-a1.y)*(b2.y-b1.y);}
double cross(geometric a1,geometric a2,geometric b1,geometric b2){return (a2.x-a1.x)*(b2.y-b1.y)-(a2.y-a1.y)*(b2.x-b1.x);}
double corner(geometric a1,geometric a2,geometric b1,geometric b2){return dot(a1,a1,b1,b2)/(dis(a1,a2)*dis(b1,b2));}
double area(geometric a1,geometric a2,geometric b1,geometric b2){return fabs(cross(a1,a2,b1,b2));}
double angle(geometric a){return atan2(a.y,a.x);}
}opt;
int n, m, tot, head=1, tail=1; double ans;
geometric data[maxn], origin, T[maxn];
struct line{
geometric A,B;double An;
line(geometric a,geometric b):A(a),B(b) {An=opt.angle(B);}
line(){}
bool operator < (const line &a)const{return An<a.An;}
geometric sdot(line a,line b){
geometric c=a.A-b.A;
double k=opt.cross(origin,b.B,origin,c)/opt.cross(origin,a.B,origin,b.B);
return a.A+a.B*k;
}
}q[maxn], p[maxn], take;
int main()
{
scanf("%d",&n);
for(int i=1;i<=n;i++)
{
scanf("%d",&m);
for(int j=1;j<=m;j++)
scanf("%lf%lf",&data[j].x,&data[j].y);
for(int j=1;j<=m;j++)
{
if(j==m)p[++tot]=line(data[j],data[1]-data[j]);
else p[++tot]=line(data[j],data[j+1]-data[j]);
}
}
sort(p+1,p+tot+1);
q[head]=p[head];
for(int i=2;i<=tot;i++)
{
while(head<tail&&opt.cross(origin,p[i].B,p[i].A,T[tail-1])<=eps)tail--;
while(head<tail&&opt.cross(origin,p[i].B,p[i].A,T[head])<=eps)head++;
q[++tail]=p[i];
if(fabs(opt.cross(origin,q[tail].B,origin,q[tail-1].B))<=eps)
{
tail--;
if(opt.cross(origin,q[tail].B,q[tail].A,p[i].A)>eps)q[tail]=p[i];
}
if(head<tail)T[tail-1]=take.sdot(q[tail-1],q[tail]);
}
while(head<tail&&opt.cross(origin,q[head].B,q[head].A,T[tail-1])<=eps)tail--;
if(tail-head>1)
T[tail]=take.sdot(q[head],q[tail]);
for(int i=head;i<=tail;i++)
{
if(i==tail)ans+=opt.cross(origin,T[i],origin,T[head]);
else ans+=opt.cross(origin,T[i],origin,T[i+1]);
}
printf("%.3lf",ans/2);
return 0;
}
|
一些例题
[ZJOI2008]瞭望塔
[HNOI2008]水平可见直线
[JLOI2013]赛车
[HNOI2012]射箭
随机增量
随机增量法
随机增量法可以用来解决最小圆覆盖。
首先,我们先思考一下这个问题:
给定平面上 n 个点,求一个半径最小的圆去覆盖这 n 个点。
我们可以先设点集 A 的最小圆覆盖为 c(A),对于一个最小覆盖圆,它肯定满足以下性质:
-
c(A) 是唯一的;
-
圆上有三个(或以上)A 中的点;
-
圆上有两个点为一条直径的两端.
其中第二条和第三条必须满足其中之一。
我们先假设目前已经求出了 i−1 个点的最小覆盖圆,在加入第 i 个点后,最小覆盖圆一定是:
额。。易证!
主要的代码部分:
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
| for(int i=2;i<=n;i++)
{
if(check(center,r,d[i]))continue;
geometric now;double nr=0;
for(int j=1;j<i;j++)
{
if(check(now,nr,d[j]))continue;
now=(d[i]+d[j])/2.0;
nr=opt.dis(d[i],d[j])/2.0;
for(int k=1;k<j;k++)
{
if(check(now,nr,d[k]))continue;
now=Center(d[i],d[j],d[k]);
nr=opt.dis(now,d[i]);
}
}
center=now;r=nr;
}
|
然后我们发现,我们似乎写了一个 O(n3) 的暴力。。其实不然。
时间复杂度的证明
由于 n 个点最多由三个点确定的最小覆盖圆,因此每个点参与确定最下覆盖圆的概率不大于 n3。
所以每一层在第 i 个点处调用下一层的概率不大于 i3。
设三个循环的时间复杂度为 T1,T2,T3:
T1(n)T2(n)T3(n)=O(n)+i=1∑ni3T2(i)=O(n)+i=1∑ni3T3(i)=O(n)
可以解得 T1=T2=T3=O(n)。
证毕。
但是这只是在数据随机的情况下,但是出题人往往不这么做,所以我们需要用 random_shuffle 函数进行扰动就很好了。
最后 n3 过百万!!(迫真)
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
| #include<bits/stdc++.h>
#define eps 1e-8
using namespace std;
const int maxn=1e5+10;
struct geometric{
double x,y;
geometric(double X=0,double Y=0):x(X),y(Y) {}
friend geometric operator + (const geometric a,const geometric b){return geometric(a.x+b.x,a.y+b.y);}
friend geometric operator - (const geometric a,const geometric b){return geometric(a.x-b.x,a.y-b.y);}
friend geometric operator * (const geometric a,double p){return geometric(a.x*p,a.y*p);}
friend geometric operator / (const geometric a,double p){return geometric(a.x/p,a.y/p);}
double dis(geometric a,geometric b){return sqrt((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y));}
double dot(geometric a1,geometric a2,geometric b1,geometric b2){return (a2.x-a1.x)*(b2.x-b1.x)+(a2.y-a1.y)*(b2.y-b1.y);}
double cross(geometric a1,geometric a2,geometric b1,geometric b2){return (a2.x-a1.x)*(b2.y-b1.y)-(a2.y-a1.y)*(b2.x-b1.x);}
double corner(geometric a1,geometric a2,geometric b1,geometric b2){return dot(a1,a1,b1,b2)/(dis(a1,a2)*dis(b1,b2));}
double area(geometric a1,geometric a2,geometric b1,geometric b2){return fabs(cross(a1,a2,b1,b2));}
double angle(geometric a){return atan2(a.y,a.x);}
geometric rotate_clockwise(geometric a,double theta){return geometric(a.x*cos(theta)-a.y*sin(theta),a.x*sin(theta)+a.y*cos(theta));}
geometric rotate_counterclockwise(geometric a,double theta){return geometric(a.x*cos(theta)+a.y*sin(theta),-a.x*sin(theta)+a.y*cos(theta));}
}opt, origin, d[maxn], st[maxn];
int n, top; double S=0, rx, ry;
int Sure(double x){return fabs(x)<eps?0:(x<0?-1:1); }
bool check(geometric a, double r, geometric b){return Sure(r-opt.dis(a, b))>=0; }
geometric Center(geometric a, geometric b, geometric c)
{
geometric mid1,mid2,cen;
double k1=0,k2=0,b1=0,b2=0;
if(a.y!=b.y)k1=-(a.x-b.x)/(a.y-b.y);
if(b.y!=c.y)k2=-(b.x-c.x)/(b.y-c.y);
mid1=(a+b)/2.0;mid2=(b+c)/2.0;
b1=mid1.y-mid1.x*k1;b2=mid2.y-mid2.x*k2;
if(k1==k2)
cen=(a+c)/2.0;
else
{
cen.x=(b2-b1)/(k1-k2);
cen.y=k1*cen.x+b1;
}
return cen;
}
int main()
{
srand(time(0));
scanf("%d",&n);
for(int i=1;i<=n;i++)
scanf("%lf%lf",&d[i].x,&d[i].y);
random_shuffle(d+1,d+n+1);
geometric center=d[1];double r=0;
for(int i=2;i<=n;i++)
{
if(check(center,r,d[i]))continue;
geometric now;double nr=0;
for(int j=1;j<i;j++)
{
if(check(now,nr,d[j]))continue;
now=(d[i]+d[j])/2.0;
nr=opt.dis(d[i],d[j])/2.0;
for(int k=1;k<j;k++)
{
if(check(now,nr,d[k]))continue;
now=Center(d[i],d[j],d[k]);
nr=opt.dis(now,d[i]);
}
}
center=now;r=nr;
}
printf("%0.10lf\n%0.10lf %0.10lf",r,center.x,center.y);
return 0;
}
|
一些例题
最小圆覆盖
[AHOI2012]信号塔
闵可夫斯基和
闵可夫斯基和
闵可夫斯基和,又称作闵可夫斯基加法,是两个欧几里得空间的点集的和,以德国数学家闵可夫斯基命名。(小知识:闵可夫斯基曾经做过爱因斯坦的老师。)
闵可夫斯基和是两个欧几里得空间的点集的和,也称为这两个空间的膨胀集,被定义为
A+B={a+b∣a∈A,b∈B}
根据闵可夫斯基和的定义,若集合元素所处代数系统满足阿贝尔群(加法可交换),则闵可夫斯基和本身也满足交换律:
A+B=B+A
(以上参考自 Baidu)
其实对于闵可夫斯基和,可以通俗的理解为,对于一个凸包 A 绕着凸包 B 转一圈:

算法实现
对于求两个点集的闵可夫斯基和,通过肉眼观察,和理性分析,我们可以得出这么一个结论:两个凸包A和B上的边一定在闵可夫斯基和中出现。
然后我们就可以先求出两个点集的凸包,然后按极角排序后求闵可夫斯基和。
求凸包:
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
| int Convexhull(geometric *p,ll l)
{
for(int i=2;i<=l;i++)
{
if(p[i].y<p[1].y)swap(p[1],p[i]);
if(p[i].y==p[1].y&&p[i].x<p[1].x)
swap(p[i],p[1]);
}
geometric k=p[1];
for(int i=1;i<=l;i++)p[i]=p[i]-k;
sort(p+2,p+l+1,cmp);
int top=0;
geometric st[maxn];
st[++top]=p[1];
for(int i=2;i<=l;i++)
{
while(top>1&&cross(st[top-1],st[top],st[top],p[i])<0)
top--;
st[++top]=p[i];
}
for(int i=1;i<=top;i++)p[i]=st[i]+k;
p[top+1]=p[1];
return top;
}
|
求闵可夫斯基和:
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
| void Minkowski()
{
for(int i=1;i<=n;i++)s1[i]=p1[i+1]-p1[i];
for(int i=1;i<=m;i++)s2[i]=p2[i+1]-p2[i];
S[++cnt]=p1[1]+p2[1];
int i=1,j=1;
while(i<=n&&j<=m)
{
cnt++;
if(cross(origin,s1[i],origin,s2[j])>=0)
S[cnt]=S[cnt-1]+s1[i++];
else S[cnt]=S[cnt-1]+s2[j++];
}
while(i<=n)
{
cnt++;
S[cnt]=S[cnt-1]+s1[i++];
}
while(j<=m)
{
cnt++;
S[cnt]=S[cnt-1]+s2[j++];
}
}
|
一些例题
[JSOI2018]战争
Pick 定理
给定顶点均为整点的多边形,其面积 S 和内部格点数目 i,边上格点数目 b 的关系为:
S=i+2b−1
其与欧拉公式,
V−E+F=2
是等价的( F 为面数,V 为顶点数,E 为边数)
(好像可以证明为什么只有五种正多面体🤔)
扫描线
扫描线是用来解决二维平面内的矩形面积并的,

主要还是用到了线段树和坐标离散化。
用线段树维护当前位置上是否有矩形即可,
题目
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
| #include<bits/stdc++.h>
#define ll long long
#define lson(p) (p<<1)
#define rson(p) (p<<1|1)
using namespace std;
const int maxn=1e5+10;
ll n,num[4*maxn],tot,cnt,ans;
struct segment{
ll Lx,Ly,Rx,Ry,v;
segment(int x1=0,int y1=0,int x2=0,int y2=0,int V=0):Lx(x1),Ly(y1),Rx(x2),Ry(y2),v(V) {}
friend bool operator < (const segment x,const segment y){
if(x.Ly==y.Ly)return x.Lx<y.Lx;
return x.Ly<y.Ly;
}
ll len(segment x,segment y){return Rx-Lx;}
}line[maxn*2];
struct Segmentree{
ll val,ls,rs,cnt;
}t[maxn<<4];
int len(int p){return num[t[p].rs]-num[t[p].ls-1];}
ll read()
{
ll x=0,f=1;char ch=getchar();
while(ch<'0'||ch>'9'){if(ch=='-')f=-1;ch=getchar();}
while(ch>='0'&&ch<='9'){x=x*10+ch-'0';ch=getchar();}
return x*f;
}
void LSH()
{
sort(num+1,num+tot+1);
tot=unique(num+1,num+tot+1)-num-1;
sort(line+1,line+cnt+1);
}
void push_up(int p)
{
if(t[p].cnt)t[p].val=len(p);
else t[p].val=t[lson(p)].val+t[rson(p)].val;
}
void build(int l,int r,int p)
{
t[p].ls=l;t[p].rs=r;
if(l==r)return;
int mid=(l+r)>>1;
build(l,mid,lson(p));
build(mid+1,r,rson(p));
}
void change(int l,int r,int p,int x,int y,int k)
{
if(x<=l&&r<=y)
{
t[p].cnt+=k;
push_up(p);
return ;
}
int mid=(l+r)>>1;
if(x<=mid)change(l,mid,lson(p),x,y,k);
if(mid<y)change(mid+1,r,rson(p),x,y,k);
push_up(p);
}
int main()
{
n=read();
for(int i=1;i<=n;i++)
{
ll x1,x2,y1,y2;
x1=read();y1=read();x2=read();y2=read();
line[++cnt]=segment(x1,y1,x2,y1,1);
line[++cnt]=segment(x1,y2,x2,y2,-1);
num[++tot]=x1;num[++tot]=x2;
}
LSH();
build(1,tot,1);
for(int i=1;i<=cnt;i++)
{
int x=lower_bound(num+1,num+tot+1,line[i].Lx)-num;
int y=lower_bound(num+1,num+tot+1,line[i].Rx)-num;
if(line[i].Ly!=line[i-1].Ly&&i!=1)
ans+=(line[i].Ly-line[i-1].Ly)*t[1].val;
change(1,tot,1,x+1,y,line[i].v);
}
printf("%lld",ans);
return 0;
}
|
窗口的星星
首先我们可以先固定住矩形的右上角使其在一定的范围内可以覆盖到星星,这时这个范围就是第一个以该星星为最下角的一个矩阵,权值即为这个星星的亮度,转化为区间最值问题。
偏序问题
二维偏序
给定 n 个二元组 (x,y),求满足条件的二元组对数。
比如逆序对,这里给出权值线段树的方法
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
| #include<bits/stdc++.h>
#define lson(p) (p<<1)
#define rson(p) (p<<1|1)
using namespace std;
const int maxn=5e5+10;
int n, a[maxn], h[maxn], size;
int k[maxn], indx[maxn];
long long ans;
struct Segment{
int ls,rs,val;
}t[maxn<<2];
void push_up(int p)
{
t[p].val=t[lson(p)].val+t[rson(p)].val;
}
void update(int l, int r, int p, int x)
{
if(l==r)
{
t[p].val++;
return;
}
int mid=(l+r)>>1;
if(x<=mid)
{
ans+=t[rson(p)].val;
update(l,mid,lson(p),x);
}
else update(mid+1,r,rson(p),x);
push_up(p);
}
int main()
{
scanf("%d",&n);
for(int i=1;i<=n;i++)
scanf("%d",&a[i]),h[i]=a[i];
sort(h+1,h+n+1);
size=unique(h+1,h+n+1)-h-1;
for(int i=1;i<=n;i++)
{
indx[i]=lower_bound(h+1,h+size+1,a[i])-h;
update(1,size,1,indx[i]);
}
printf("%lld\n",ans);
return 0;
}
|
三维偏序
给定 n 个三元组 (x,y,z),求满足条件的对数。
先对 x 排序,将其转化为二维偏序,然后用 CDQ 分治,将 y 排序,用树状数组维护 z
陌上花开
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
| #include<bits/stdc++.h>
const int maxn=1e5+10;
using namespace std;
int n,cnt=1,Max,Ans[maxn];
struct node{
int a,b,c,t,val;
}d[maxn];
struct Segmentree{
int ls,rs,val;
}t[maxn<<3];
void push_up(int p)
{
t[p].val=t[t[p].ls].val+t[t[p].rs].val;
}
bool cmp1(node x,node y)
{
if(x.a==y.a)
{
if(x.b==y.b)
return x.c<y.c;
else return x.b<y.b;
}
else return x.a<y.a;
}
bool cmp2(node x,node y)
{
if(x.b==y.b)
return x.c<y.c;
else return x.b<y.b;
}
int Insert(int l,int r,int p,int x,int k,int& sum)
{
if(p==0)p=++cnt;
if(l==r)
{
sum+=t[p].val;
t[p].val+=k;
return p;
}
int mid=(l+r)>>1;
if(x<=mid)
t[p].ls=Insert(l,mid,t[p].ls,x,k,sum);
else
{
sum+=t[t[p].ls].val;
t[p].rs=Insert(mid+1,r,t[p].rs,x,k,sum);
}
push_up(p);
return p;
}
void cdq(int l,int r)
{
if(l==r)return;
int mid=(l+r)>>1;
cdq(l,mid);
cdq(mid+1,r);
sort(d+l,d+mid+1,cmp2);
sort(d+mid+1,d+r+1,cmp2);
cnt=1;
int sum,j=l;
for(int i=mid+1;i<=r;i++)
{
while(d[i].b>=d[j].b&&j<=mid)
{
Insert(1,Max,1,d[j].c,d[j].t,sum);
j++;
}
sum=0;
Insert(1,Max,1,d[i].c,0,sum);
d[i].val+=sum;
}
for(int i=1;i<=cnt;i++)
t[i].ls=t[i].rs=t[i].val=0;
}
int main()
{
scanf("%d%d",&n,&Max);
int N=n;
for(int i=1;i<=n;i++)
scanf("%d%d%d",&d[i].a,&d[i].b,&d[i].c);
sort(d+1,d+n+1,cmp1);
int tot=0;
for(int i=1,len=1;i<=n;i++)
{
if(d[i].a!=d[i+1].a||d[i].b!=d[i+1].b||d[i].c!=d[i+1].c)
{
tot++;
d[tot]=d[i];
d[tot].t=len;
len=1;
}
else len++;
}
n=tot;
cdq(1,n);
for(int i=1;i<=n;i++)
Ans[d[i].val+d[i].t-1]+=d[i].t;
for(int i=0;i<N;i++)
printf("%d\n",Ans[i]);
return 0;
}
|
n 维偏序
四维的话可以考虑 CDQ 套 CDQ,更高的话直接 KD-tree。
数值积分
数值积分,是用来求定积分的近似值。
这里我们只阐述一种数值积分的求法,使用 Simpson 公式。
Simpson公式
Simpson 公式就是在积分区间 [a,b] 中去找三个点 a,b 和 m=(a+b)/2 ,计算其原函数在此处的值,然后用抛物线来拟合原函数。
tips:幂函数的积分公式:
∫xαdx=α+11xα+1+C(C为常数项)
尝试推导一下公式🤔
设 f(x) 为原函数,g(x)=Ax2+Bx+C 为拟合后的函数,有:
∫abf(x)dx≈∫abAx2+Bx+C=3A(b3−a3)+2B(b2−a2)+C(a−b)=6(b−a)(2A(b2+ab+a2)+3B(b+a)+6C)=6(b−a)(2Ab2+2Aab+2Aa2+3Bb+3Ba+6C)=6(b−a)(Aa2+Ba+C+Ab2+Bb+C+4(A(2a+b)2+B(2a+b)+C))=6(b−a)(f(a)+f(b)+4f(2a+b))
最终 Simpson 公式为:
∫abf(x)dx≈6(b−a)(f(a)+f(b)+4f(2a+b))
1
2
3
4
| double simpson(double l, double r)
{
return (r - l)*(f(l) + f(r) + 4*f((l+r)/2))/6;
}
|
有一个结论:Simpson 公式的误差为
−901(2r−l)5f(4)(ξ)
其中 ξ 为区间 [l,r] 中的某个值。
是不是还没说数值积分学这玩意干嘛,很明显是解决积分问题的,想想上面我们说的黎曼积分的定义,其精确度很明显是由区间分割决定的,如何区间分割呢?
自适应Simpson法
现在来解决精度问题,让其实现自动控制分割区间的大小。
Simpson 公式是通过一个二次函数去拟合原函数的,也就是说我们当前分割的区间对应的函数值越接近一个二次函数图像,误差就越小。
也就是说我们在计算时需要判断当前区间是否接近一个二次函数,判断过程如下:
首先将当前段直接代入求积分,在将当前这一段分成两个区间,求这两段的积分,如果当前段的积分值和分割成两段之后的积分之和相差很小的话就可以直接计算了否则递归。
有一个结论:三点 Simpson 与分成两个子区间后两个子区间 Simpson 和差值是原来绝对误差的 151(我不太会证)
1
2
3
4
5
6
7
8
9
10
11
| double auto_simpson(double l, double r, double eps, double fx)
{
double mid = (l+r)/2;
double L = simpson(l, mid);
double R = simpson(mid, r);
if(fabs(L + R - fx) <= 15*eps)
return L + R + (L + R - fx)/15;
else
return auto_simpson(l, mid, eps/2, L)+
auto_simpson(mid, r, eps/2, R);
}
|
给定积分:
∫LRax+bcx+ddx
保留至小数点后六位。
直接使用公式即可。
或者直接积出来,直接暴切
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
| #include <bits/stdc++.h>
using namespace std;
double a, b, c, d;
double f(double x)
{
return (c*x + d)/(a*x + b);
}
double simpson(double l, double r)
{
return (r - l)*(f(l) + f(r) + 4*f((l+r)/2))/6;
}
double auto_simpson(double l, double r, double eps, double fx)
{
double mid = (l+r)/2;
double L = simpson(l, mid);
double R = simpson(mid, r);
if(fabs(L + R - fx) <= 15*eps)
return L + R + (L + R - fx)/15;
else
return auto_simpson(l, mid, eps/2, L)+
auto_simpson(mid, r, eps/2, R);
}
int main()
{
double l, r;
scanf("%lf%lf%lf%lf%lf%lf", &a, &b, &c, &d, &l, &r);
printf("%.6lf", auto_simpson(l, r, 1e-6, simpson(l, r)));
return 0;
}
|
给定积分:
∫0∞xxa−xdx
保留小数点后5位,会出现积分发散的情况。
好像这个式子是一个不可积分函数,只能用 Simpson ,(也只会 Simpson ),但是下界是0,上界是 ∞,需要找到式子的一些性质。
可以证明:
-
a<0 时原积分发散
∵xa−x=xa−x2∴a−x2<0,xxa−x2=xxx2−a1∵x→0,x2→0,x1→∞∴0<x<1,xk→0,k→∞∴x→0,f(x)→∞
-
a>0 时原积分收敛
∵xxa−x2=xxx2−a1∴x→∞,f(x)→0
因为在 a>0 的时候收敛所以右边界不用设太大,可以自己打表试一下。
特判 a<0,在 [1e−8,20] 作为积分范围即可。
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
| #include <bits/stdc++.h>
using namespace std;
double a;
double f(double x)
{
return pow(x, a/x - x);
}
double simpson(double l, double r)
{
return (r - l)*(f(l) + f(r)+ 4*f((l+r)/2))/6;
}
double auto_simpson(double l, double r, double eps, double fx)
{
double mid = (l+r)/2;
double L = simpson(l, mid);
double R = simpson(mid, r);
if(fabs(L+R-fx) <= 15*eps)
return L + R + (L + R - fx)/15;
else
return auto_simpson(l, mid, eps/2, L) +
auto_simpson(mid, r, eps/2, R);
}
int main()
{
scanf("%lf", &a);
double l = 1e-9;
double r = 20;
if(a < 0)
printf("orz");
else
printf("%.5lf", auto_simpson(l, r, 1e-8, simpson(l, r)));
return 0;
}
|
给定一个由圆台和圆锥构成的组合体,给定一个角度求求其在平面上的投影面积。
体现了自适应辛普森法的一个常见用途,用来求曲边图形的面积 。
先来考虑投影的形状,首先一个圆的投影肯定是一个大小相同的圆,圆锥的投影就是一个圆加上了一个点,一级这个点和圆的两条切线,圆台的投影就是两个圆加上它们的公切线。
连起来的话就是一个非常鬼畜的东西。

然后暴力即可(画的好像不太标准\kk)
是一个封闭图形,并且将其看作一个函数直接求积分即可。
再去观察图形,此时这棵树的投影已经变为了若干个圆弧加上若干个梯形和三角形。
既然是求积分的话而且还存在圆弧弧就需要用的 Simpson 公式了。
需要用梯形腰的左右两个端点作为一段函数的定义域。
然后就是高度为 h 的物体投影到水平面上长度会变为 tanαh,来确定横坐标。
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
| #include <bits/stdc++.h>
#include <pthread.h>
#define dwd 1919810.114514
#define ochen 114514.1919810
using namespace std;
const int N = 510;
int n;
double alpha;
double H, Tan;
struct geometric{
double x,y;
geometric(double X=0,double Y=0):x(X),y(Y) {}
friend geometric operator + (const geometric a,const geometric b){return geometric(a.x+b.x,a.y+b.y);}
friend geometric operator - (const geometric a,const geometric b){return geometric(a.x-b.x,a.y-b.y);}
friend geometric operator * (const geometric a,double p){return geometric(a.x*p,a.y*p);}
friend geometric operator / (const geometric a,double p){return geometric(a.x/p,a.y/p);}
double dis(geometric a,geometric b){return sqrt((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y));}
double dot(geometric a1,geometric a2,geometric b1,geometric b2){return (a2.x-a1.x)*(b2.x-b1.x)+(a2.y-a1.y)*(b2.y-b1.y);}
double cross(geometric a1,geometric a2,geometric b1,geometric b2){return (a2.x-a1.x)*(b2.y-b1.y)-(a2.y-a1.y)*(b2.x-b1.x);}
double corner(geometric a1,geometric a2,geometric b1,geometric b2){return dot(a1,a2,b1,b2)/(dis(a1,a2)*dis(b1,b2));}
double area(geometric a1,geometric a2,geometric b1,geometric b2){return fabs(cross(a1,a2,b1,b2));}
double angle(geometric a){return atan2(a.y,a.x);}
geometric rotate_counterclockwise(geometric a,double theta){return geometric(a.x*cos(theta)-a.y*sin(theta),a.x*sin(theta)+a.y*cos(theta));}
geometric rotate_clockwise(geometric a,double theta){return geometric(a.x*cos(theta)+a.y*sin(theta),-a.x*sin(theta)+a.y*cos(theta));}
}opt;
struct circle{
geometric p;
double r;
circle(geometric _p = 0, double _r = 0): p(_p), r(_r) {}
};
circle c[N];
struct line{
geometric a, b;
line(geometric _a = 0, geometric _b = 0):a(_a), b(_b) {}
double calc(double x)
{
double k = (b.y-a.y)/(b.x-a.x);
double b = a.y-k*a.x;
return k*x+b;
}
}seg[N];
double f(double x)
{
double sum = 0;
for(int i = 1; i <= n; i++)
{
if(x < c[i].p.x + c[i].r && x > c[i].p.x - c[i].r)
sum = max(sum, sqrt(c[i].r*c[i].r-(x-c[i].p.x)*(x-c[i].p.x)));
}
for(int i = 1; i < n; i++)
{
if(x > seg[i].a.x && x < seg[i].b.x)
sum = max(sum, seg[i].calc(x));
}
return sum;
}
double simpson(double l, double r)
{
return (r-l)*(f(l) + f(r) + 4*f((l+r)/2))/6;
}
double auto_simpson(double l, double r, double eps, double fx)
{
double mid = (l+r)/2;
double L = simpson(l, mid);
double R = simpson(mid, r);
if(fabs(L + R - fx) <= 15*eps)
return L + R + (L + R - fx)/15;
else
return auto_simpson(l, mid, eps/2, L) + auto_simpson(mid, r, eps/2, R);
}
int main()
{
scanf("%d%lf", &n, &alpha);
Tan = 1.0/tan(alpha);
n = n + 1;
for(int i = 1; i <= n; i++)
{
double h;
scanf("%lf", &h);
c[i].p.x = h*Tan;
c[i].p.x += c[i-1].p.x;
}
for(int i = 1; i < n; i++)
{
double r;
scanf("%lf", &r);
c[i].r = r;
}
c[n].r = 0;
for(int i = 1; i < n; i++)
{
double x1 = c[i].p.x;
double x2 = c[i+1].p.x;
double r1 = c[i].r;
double r2 = c[i+1].r;
double a1 = r1*(r1-r2)/(x2-x1);
double a2 = r2*(r1-r2)/(x2-x1);
seg[i] = line(geometric(x1+a1, sqrt(r1*r1-a1*a1)),geometric(x2+a2, sqrt(r2*r2-a2*a2)));
}
double l = c[1].p.x - c[1].r;
double r = c[n].p.x - c[n].r;
for(int i = 1; i <= n; i++)
{
l = min(l, c[i].p.x - c[i].r);
r = max(r, c[i].p.x + c[i].r);
}
printf("%.2lf", 2*auto_simpson(l, r, 1e-6, simpson(l, r)));
return 0;
}
|
反演变换
前言
反演变换适用于题目中出现多个圆或直线之间的相切关系的情况,反演变换可以有效简便的解决这类问题。
定义
设在平面内给定一点O和常数k,对于平面内任意一A,确定A′,使A′为直线OA上一点,并且OA⋅OA′=k,我们称这种变换为反演变换,点O即为反演中心A′为A关于点O的反演点。
反演变换本质上是属于平移,旋转,反射一类的几何变换。
以O点为圆心,∣k∣为半径作圆,就得到了一个圆的反演变换:
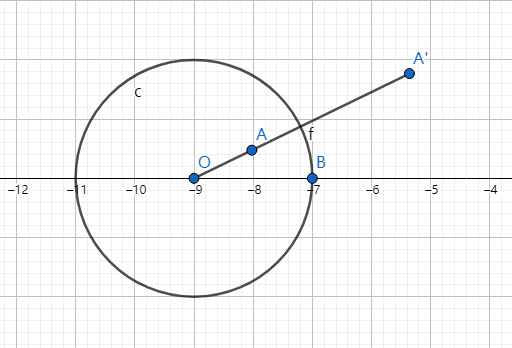
接下来讨论其相关性质。
性质
性质1
反演变换可逆
对于定义里的描述,A′为A关于O点的反演点,同时就有A是关于A′的反演点。
性质2
- 在圆内的点其反演点一点在圆外
- 在圆外的点其反演点一定在圆内
- 在圆上的点的反演点与其在同一个位置
观察图形很容易得到的性质。
接下来引入一些几何图形关于圆的反演。
性质3
-
过反演中心的直线其反演后为自身
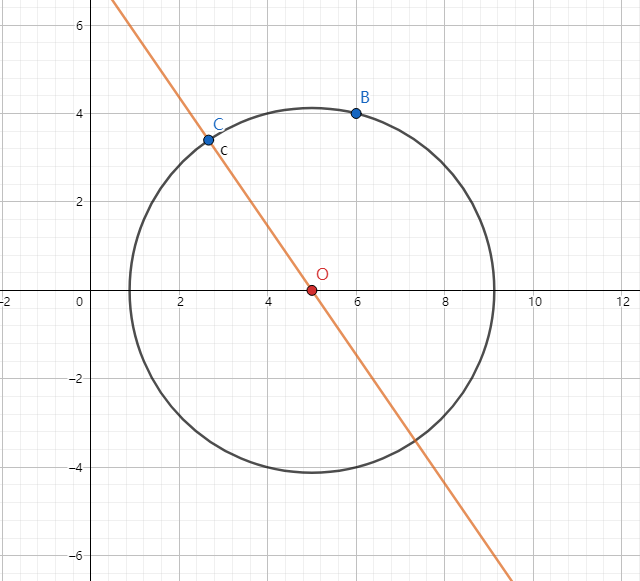
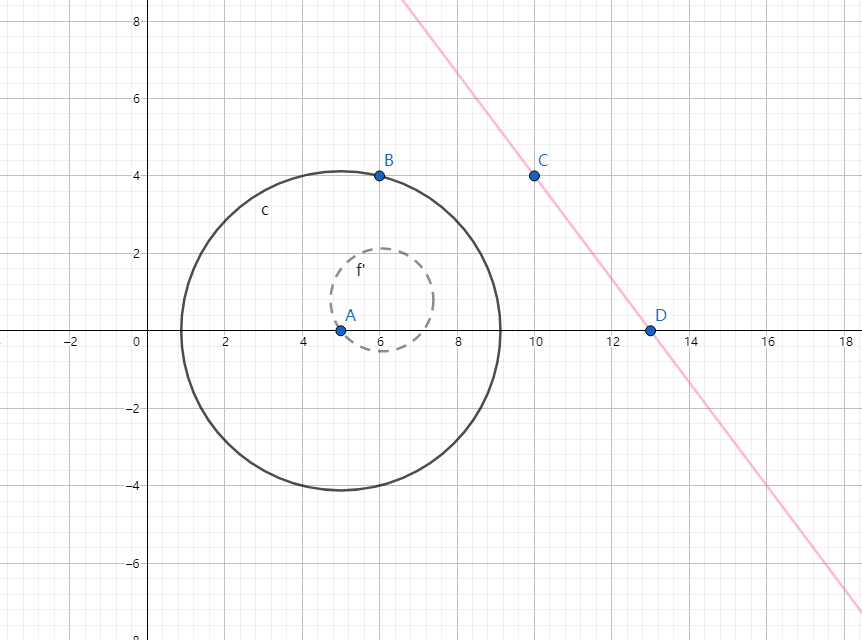
也许需要证明?也挺好推的,设反演中心为原点,直线解析式为y=k1x+b,反演得到的线段为y=k2x,可以推得交点为k2−k1b,设为c,再设交点的反演点为(x,k2x),再根据反演的定义得(c2+k22c2)(x2+k2x2)=r4,拆开后去掉常数项,会得到一个xy+y2=C(C∈R)的圆的方程。
性质4
证明的话依然是利用解析式去证明,和上个性质证明过程没有太大区别。
性质5
两条直线或曲线的夹角大小在反演变换下是不变的。
体现了反演的反向保角性
性质6
两个圆相切,它们的反演图形也相切
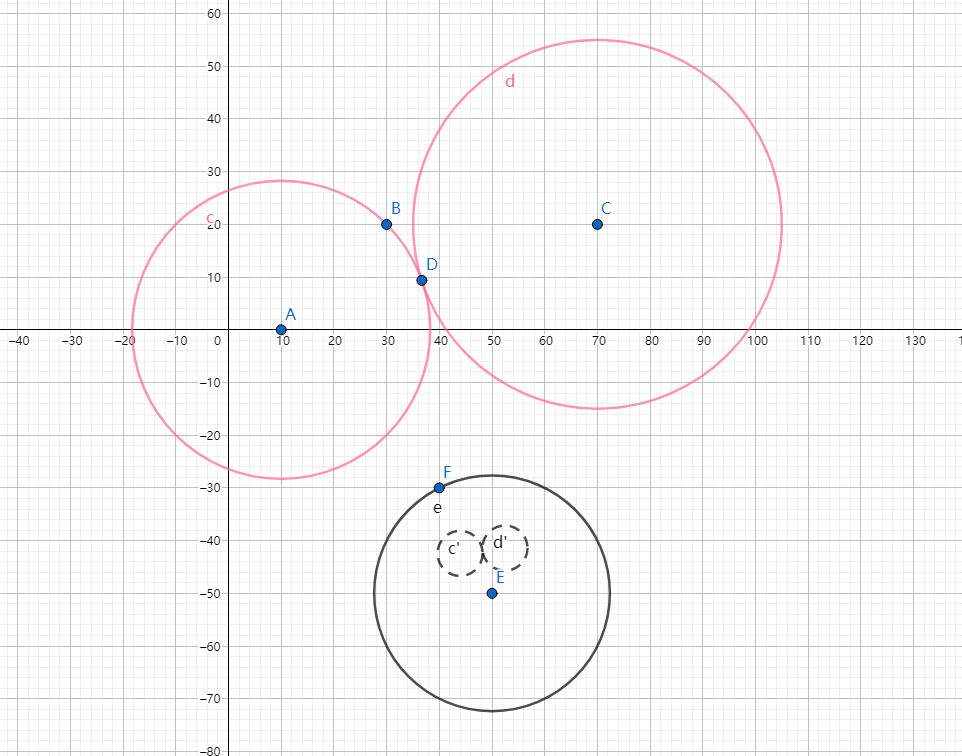
(易证)
计算几何杂项
本来想写到前面的,结果写到这里了
判断精度
精度问题是计算几何非常恶心的一个地方,有时候一个非常合适的精度可以决定你是否能 A 掉这个题
1
2
3
4
5
6
7
8
| const double eps=1e-10;
int sure(double x)
{
return fabs(x)<eps?0:x<0?-1:1;
}
|
点
1
2
3
| struct Point{
double x,y;
};
|
向量
记录起点和终点
1
2
3
4
5
6
| struct Vector{
Point a,b;
};
|
四则运算
1
2
3
4
| friend geometric operator + (const geometric a,const geometric b){return geometric(a.x+b.x,a.y+b.y);}
friend geometric operator - (const geometric a,const geometric b){return geometric(a.x-b.x,a.y-b.y);}
friend geometric operator * (const geometric a,double p){return geometric(a.x*p,a.y*p);}
friend geometric operator / (const geometric a,double p){return geometric(a.x/p,a.y/p);}
|
距离
1
2
| double dis(Point a, Point b){return sqrt((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y)); }
|
点积
1
| double dot(Point a1,Point a2,Point b1,Point b2){return (a2.x-a1.x)*(b2.x-b1.x)+(a2.y-a1.y)*(b2.y-b1.y);}
|
叉积
1
2
| double cross(geometric a1, geometric a2, geometric b1, geometric b2){return (a2.x-a1.x)*(b2.y-b1.y)-(a2.y-a1.y)*(b2.x-b1.x); }
|
向量夹角
1
| double corner(geometric a1,geometric a2,geometric b1,geometric b2){return dot(a1,a1,b1,b2)/(dis(a1,a2)*dis(b1,b2));}
|
两向量围成的四边形面积
1
2
| double area(geometric a1, geometric a2, geometric b1, geometric b2){return fabs(cross(a1, a2, b1, b2)); }
|
极角
1
| double angle(geometric a){return atan2(a.y,a.x);}
|
逆时针旋转
1
2
| geometric rotate_counterclockwise(geometric a, double theta){return geometric(a.x*cos(theta)-a.y*sin(theta), a.x*sin(theta)+a.y*cos(theta)); }
|
顺时针旋转
1
| geometric rotate_clockwise(geometric a,double theta){return geometric(a.x*cos(theta)+a.y*sin(theta),-a.x*sin(theta)+a.y*cos(theta));}
|
直线
点加方向向量
1
2
3
4
5
6
7
8
9
10
11
12
13
14
| struct line{
geometric A,B;double An;
line(geometric a,geometric b):A(a),B(b) {An=opt.angle(B);}
line(){}
bool operator < (const line &a)const{return An<a.An;}
geometric sdot(line a,line b){
geometric c=a.A-b.A;
double k=opt.cross(origin,b.B,origin,c)/opt.cross(origin,a.B,origin,b.B);
return a.A+a.B*k;
}
};
|
三维计算几何
1
2
3
4
5
6
7
8
9
10
11
| struct geometric{
double x,y,z;
geometric(double X=0,double Y=0,double Z=0):x(X),y(Y),z(Z) {}
friend geometric operator + (const geometric a,const geometric b){return geometric(a.x+b.x,a.y+b.y,a.z+b.z);}
friend geometric operator - (const geometric a,const geometric b){return geometric(a.x-b.x,a.y-b.y,a.z-b.z);}
friend geometric operator * (const geometric a,double p){return geometric(a.x*p,a.y*p,a.z*p);}
friend geometric operator / (const geometric a,double p){return geometric(a.x/p,a.y/p,a.z/p);}
double dis(geometric a,geometric b){return sqrt((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y)+(a.z-b.z)*(a.z-b.z));}
double dot(geometric a1,geometric a2,geometric b1,geometric b2){return (a2.x-a1.x)*(b2.x-b1.x)+(a2.y-a1.y)*(b2.y-b1.y)+(a2.z-a1.z)*(b2.z-b1.z);}
geometric cross(geometric a1,geometric a2,geometric b1,geometric b2){geometric a,b;return geometric(a.y*b.z-b.y*a.z,b.x*a.z-a.x*b.z,a.x*b.y-b.x*a.y);}
};
|
平面
1
2
3
4
5
6
7
8
9
| truct plane{
geometric a,b,c;
plane(geometric A=origin,geometric B=origin,geometric C=origin):a(A),b(B),c(C) {}
geometric normal(){return opt.cross(p[v[0]],p[v[1]],p[v[0]],p[v[2]]);}
double area(){return fabs(opt.dis(origin,opt.cross(a,b,a,c)))/2.0;}
};
|
计算几何板子
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
| namespace Geometric
{
#define eps 1e-8
struct Point
{
double x, y;
Point(double x = 0, double y = 0) : x(x), y(y) {}
friend Point operator + (Point a, Point b) {return Point(a.x + b.x, a.y + b.y);}
friend Point operator - (Point a, Point b) {return Point(a.x - b.x, a.y - b.y);}
friend Point operator * (Point a, double b){return Point(a.x * b, a.y * b);}
friend Point operator / (Point a, double b){return Point(a.x / b, a.y / b);}
}origin;
#define Vector Point
double dis(Point a, Point b){return sqrt((a.x - b.x) * (a.x - b.x) + (a.y - b.y) * (a.y - b.y));}
double len(Vector v){return dis(origin, v);}
double dot(Vector a, Vector b){return a.x * b.x + a.y * b.y;}
double cross(Vector a, Vector b){return a.x * b.y - b.x * a.y;}
double corner(Vector a, Vector b){return dot(a, b) / (len(a) * len(b));}
double area (Vector a, Vector b){return cross(a, b) / 2.0;}
double angle(Vector v){return atan2(v.y, v.x);}
Vector rotate_counterclockwise(Vector v, double t)
{return Vector(v.x * cos(t) - v.y * sin(t), v.x * sin(t) + v.y * cos(t));}
Vector rotate_clockwise(Vector v, double t)
{return Vector(v.x * cos(t) + v.y * sin(t), v.y * cos(t) - v.x * sin(t));}
struct line
{
Point A; Vector B; double An;
line(Point a = 0, Vector b = 0) : A(a), B(b) {An = atan2(B.y, B.x);}
friend bool operator < (line a, line b){return a.An < b.An;}
};
Point sdot(line a, line b){Point c = a.A - b.A;double k = cross(b.B, c) / cross(a.B, b.B);return a.A + a.B * k;}
int sure(double x){return fabs(x) < eps ? 0 : (x > 0 ? 1 : -1);}
}
|
最后推荐两个画图工具desmos和Geogebra!